Aerospace Micro-Lesson #78
In This Section
Hovercraft, Hydrofoils, and Ornithopters
When one thinks of flying vehicles, one thinks usually of airplanes, helicopters, and balloons. But there are other types of vehicle that fly. A hovercraft flies on a cushion of higher-pressure air underneath it. A hydrofoil does fly on wings, but the wings are embedded in water rather than in air. A third type of flying machine, an ornithopter, is more like a conventional airplane but its wings flap, providing propulsion as well as lift. This lesson explores these unusual flying machines.
Next Generation Science Standards (NGSS):
- Disciplinary: Engineering Design
- Crosscutting Concept: Structure and Function
- Science & Engineering Practice: Asking Questions and Defining Problems
GRADES K–2
A hovercraft is an unusual type of flying machine. It is a type of amphibious vehicle, meaning that it can travel on both land and water, but it is actually a flying machine because it travels on a cushion of air above the land or water. The idea for an air-cushion vehicle was first thought of by Sir Christopher Cockerell in 1955. He built a working model, improved upon it, and by 1959 the machine worked well enough that it could cross the English Channel between England and France. Although that first hovercraft was only large enough to carry a crew of three, many variations since then have been used as ferries, recreational vehicles, and even for military operations. (The picture on the previous page is of Cockerell pushing his hovercraft prototype, from The Engineer.)
Another type of flying machine, one that has more in common with airplanes, is the hydrofoil. The hydrofoil is a boat that has fins like an airplane’s wings, but the fins fly through the water rather than through the air. The lift on the fins allows the boat to ride above the surface of the water. With the friction of the water only pushing against the small fins (or foils), rather than the entire surface of the boat’s hull, the boat is able to reach higher speeds with less power and give a smoother ride. As early as 1898 inventors were experimenting with designs for how to shape and place the foils for best results. The famous Alexander Graham Bell, inventor of the telephone, became interested in the idea and created a hydrofoil boat; there is a model of his HD-4 at the Smithsonian, while a full scale replica is at the Alexander Graham Bell National Historic Site. (The picture is from National Library of Scotland of the 1919 HD-4, which set a speed record unbroken for 10 years.)
A third type of flying machine, the ornithopter, can fly at any altitude rather than being restricted to just above the land or water. Unlike the airplanes we are accustomed to, the ornithopter has wings that flap like a bird’s wings. The first designs for ornithopters, from the 1600s, envisioned that they would carry one person who would power them using his own muscles. The problem with humans trying to fly like a bird or a bat is that our bodies are not as light as those of birds or bats and our muscles become tired very quickly when trying to flap the wings of a machine to keep it in flight. Many early scientists and inventors sketched ideas for how to make such a flying machine, including Leonardo da Vinci. The National Air and Space Museum has a model that is based on one of da Vinci’s drawings. In modern times, people have built small ornithopters as model aircraft; these models actually fly, although they are not big enough to carry a practical load. A problem with a large ornithopter is that the wings, which carry the weight of the aircraft, are moving parts; the combination of motion and heavy loads causes them to wear out quickly.
GRADES 3–5
A hovercraft works using two separate effects. The hovering is created by a fan pushing air down into a space underneath the hovercraft, increasing the air pressure there. A skirt around the hovercraft prevents the air from escaping too quickly for the fan to keep up. The increased air pressure pushes up on the craft, lifting it off of the ground. A second fan blows air horizontally out the back of the craft, pushing it forward. This latter effect is an example of Newton’s Third Law, which states in plain English that if I push on something, it will push back on me. The fan pushes on the air and the air pushes back on the fan, making it (and the craft that it is attached to) move in the opposite direction. One result of the way that a hovercraft works is that it has no brakes: the cushion of air gives very little friction (an advantage when trying to move forward quickly) and the designer needs to figure out another way to slow the craft down.
Half a century ago, people built large hovercraft to carry things across short stretches of water. With more speed than a boat, no need for the docks that regular boats needed for mooring, and the ability to move over both land and water, a hovercraft offered some advantages over a boat for ferrying people and cargo. For several years a company operated hovercraft to carry passengers, cars, and cargo across the English Channel between England and France. One of the drawbacks with hovercraft, though, was the loud noise it made when it operated. Another problem was the amount of fuel needed. The rising cost of fuel made the commercial use of the original hovercraft to cross the English Channel a poor business prospect, especially once the Channel Tunnel was completed. But other air-cushion vehicles have been used in various ways. Military uses include amphibious assaults, patrols, and movement in marshy areas. The idea has also been applied to other types of transportation. Air-cushion trains (or hovertrains), have been under design in several countries, although none are in commercial use as yet. Air-cushion pallets that can help warehouse and factory workers shift heavy loads. On a very small scale, the commercial air hockey game uses an air cushion to allow the “puck” to move with almost no friction.
There are directions for creating your own tabletop model of a hovercraft available on various websites such as Steve Spangler Science.
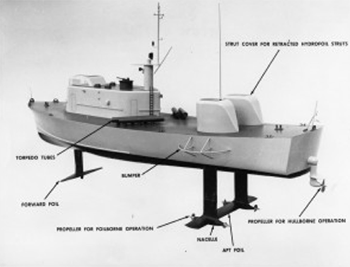
Hydrofoil boats were used by the Navy as patrol boats because of their speed and flexibility. One of the first was the U.S.S. High Point in 1962, seen on the next page.
Since that time, the use of foils has been applied to commercial ferry boats, speed boats and other recreational craft—includingstanding paddle boards—and even waterbikes. The attraction is obvious; the lowered drag and increased speed help cut costs for businesses and increase the thrills for sports fans.
 |
 |
The desire to fly like a bird has always attracted people and model aviators are not a new development. Besides the typical model airplanes, there are also model ornithopters and even an Ornithopter Society with its own website. For those interested in making their own models, this is a good starting place. It offers tips on using ready-made kits as well as experimenting with creating your own designs. Airports are even using robotic birds to scare away real birds and prevent bird strikes to aircraft.
GRADES 6–8
If a student wants to make a hovercraft, he or she can find instructions on the Internet for both larger and smaller versions. Many students build hovercraft for Science Fair projects, but your writer would discourage this for a few reasons. The Science Fair is for scientific investigations; building a hovercraft is an exercise in engineering. Science Fair projects are graded on originality; indeed, coming up with an original idea is one of the harder parts of a Science Fair project. If one can figure out some scientific question that one can investigate by building a hovercraft, that would be fine; simply building a hovercraft as the project itself should not be done.
The principle behind the working of a hovercraft is very simple: a fan blows air into a space underneath the vehicle, increasing its pressure slightly. A “skirt” around the edge of the vehicle keeps the air from getting out too quickly. The extra pressure of the air under the vehicle lifts it up off of the ground, allowing it to fly. This raises an obvious question: how much extra pressure do you need?
The answer, of course, is “it depends.” How big is the vehicle? How heavy is it (including cargo)? The pressure needed is simply the ratio of the vehicle’s weight to its area:
If you want something that can lift 2,000 pounds and can build it in the shape of a circle ten feet across (five-foot radius), then the pressure that you will need is
While this does not appear to be much, it is actually quite a bit. Multiplying it by 144 in2/ft2 translates it to 25 pounds per square foot. If the vehicle’s skirt is a foot high, then the air pressing outward on it will exert a force of 100 pounds along every four feet of length. Making the skirt shorter will reduce the force on it but will also make the hovercraft ride lower to the ground, making it need a smoother path.
In the commercial world, pressures are often expressed as “inches of water gauge” or simply “inches of water.” One inch of water is the pressure exerted by a column of water an inch high. (A “gauge pressure” is a difference in pressure, usually the difference from atmospheric pressure, as one measures with a pressure gauge. This is different from an “absolute pressure,” which is the pressure relative to an absolute vacuum.) With the density of water being 62.4 pounds per cubic foot or 0.036 pounds per cubic inch, one inch of water gauge is equal to 0.036 psi of pressure.
With this conversion factor, one can see that a circular hovercraft 10 feet in diameter lifting 2,000 pounds will exert a pressure of five inches of water gauge. If the hovercraft is hovering over water, it will be sitting in a depression five inches below the nominal water surface level.
GRADES 9–12
The BBC television show “Top Gear” had an episode in which the three hosts converted a van to a hovercraft, with comical results. You may be interested in a four-minute clip or an eight-minute clip showing them running their “hovervan” on a canal, a thirty-second clip showing them trying to park it, or the full thirty-minute episode. The videos show a few disadvantages of a hovercraft: fuel economy, noise, and a lot of water spray.
A hydrofoil is shaped like a wing (because it is a wing) and is attached to the keel of a boat. As the boat moves forward, the water flowing over the hydrofoil generates lift, just the air flowing over the wings of an airplane generates lift. The lift raises part of the boat’s hull out of the water, reducing the drag on the boat and allowing the boat’s propeller to move it forward faster. The higher speed causes the hydrofoil to generate even more lift, raising the hull farther out of the water and reducing the drag further.
The lift force on a wing is calculated as the product of the wing’s dynamic pressure, reference area, and lift coefficient. The lift coefficient is a function of the wing’s shape; the reference area is a function of its size; and the dynamic pressure is a function of the airplane’s speed through the air (or the boat’s speed through the water) and the density of the air (or water). These are combined using these formulae:
where
- L is the lift force on the wing or hydrofoil
- q is the dynamic pressure of the flow over the wing or hydrofoil
- s is the wing’s or hydrofoil’s reference area
- CLis the wing’s or hydrofoil’s lift coefficient
- r is the density of the air or water flowing over the wing or hydrofoil
- V is the speed of the wing or hydrofoil through the air or water
Comparing the performance of a hydrofoil on the keel of a boat to the performance of a wing on an airplane is instructive. The boat will travel at some tens of miles per hour; the airplane will fly at some hundreds of miles per hour, a ratio of speeds of about a factor of ten. The density of water is about 62 lbm/ft3or 1,000 Kg/m3 while the density of air at sea level is 0.076 lbm/ft3or 1.225 Kg/m3, a ratio of about 800 to one. (At high altitudes, the density of the air is even lower, making the ratio of densities even greater.) Combining these ratios tells us to expect that the dynamic pressure “q” for a typical hydrofoil will be larger than that for a typical airplane wing by a factor of about eight. This is not at all exact but is about the right order of magnitude; the typical ratio will not be 0.8 or 80 (unless the airplane wing is flying at high altitudes). The lift coefficients of the wing and hydrofoil will be about the same. To generate the same lift force, then, the hydrofoil’s reference area can be smaller by about a factor of eight, or its linear dimension can be smaller by about a factor of three.
The idea of human-powered flight imitating that of birds, as an ornithopter does, is probably as old as humanity. It suffers, however, from a problem of scale. Consider a body of some shape—any shape, but in our case a shape that we are trying to make fly. If you double its size, each of its dimensions grows by a factor of two. Its surface area, or any two-dimensional area associated with it, will grow by a factor of four. Its volume, and with the volume the mass, will grow by a factor of eight. This causes a problem with trying to make the larger object fly: the lift force, which is proportional to a reference area, increases by a factor of four while the weight that the lift force is opposing has increased by a factor of eight. It is therefore twice as difficult to lift the larger body off the ground as it is the smaller body. There is a reason that birds tend to be quite small.
Sixty Years Ago in the Space Race:
January 28, 1959: An American Nike-Cajun sounding rocket launched an inflatable twelve-foot test sphere to an altitude of 75 miles as part of the Beacon flight program.